Previous blogs have introduced the phenomenon of resonance. In fact, most objects can resonate at more than one frequency and in multiple modes of vibration. This blog will concentrate on resonance at multiples of the the fundamental frequency. These are “harmonics” of the fundamental frequency.
A good model to demonstrate the principle of resonance at multiples of the fundamental frequency is a string as from a stringed instrument. The string, of a particular length and under a particular tension, has a fundamental resonance at the frequency which sets up a 1/2 wave-length vibratory motion within the string as shown below. That frequency is called the “fundamental” because the string will not have a resonant at any lower frequency.
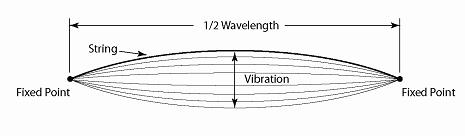
The resonant freqency of the string can, of course, be changed by changing its tesion or its length. In string instruments, the strings are first tuned at the fundamental frequency. The player then shortens the strings using his fingers to, in effect, shorten the string and produce higher and higher frequency notes. The string under a certain tension and of a certain length can not resonate at a frequency lower than its fundamental 1/2 wave length frequency. It can, however, resonate at a higher frequency.
At a frequency double that of the fundamental, the string, still at the same tension and length will also resonate. In fact, the string will resonate at any frequency that produces vibration at an integer (1, 2, 3, etc) multiple of the 1/2 wave length fundamental frequency as shown below. This effect can be easily demonstrated using a jump rope excited by two people with some manual dexterity.
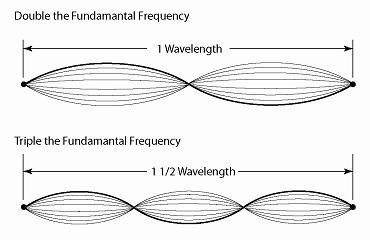
In the case of a string, both ends of the string are “fixed” meaning held in a fixed position which confines resonance to multiples of 1/2 wavelength.
Solid shapes, liquid columns and air columns, however, follow a somewhat different set of rules. An organ pipe is often used as a model in this case. The “fundamental” resonant frequency of an organ pipe with both ends open (one “open” end being comprised of whatever mechanism is used to excite the pipe) occurs when the column of air within the pipe is 1/2 wavelength long. The next resonant frequency occurs when the pipe contains a full wavelength within the confined air column as shown below.
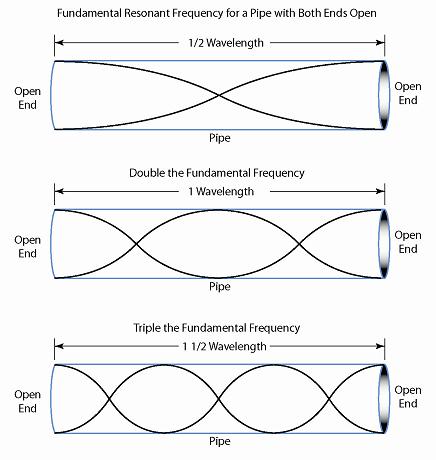
Just like the string, the resonant frequency of the pipe can be increased by making it shorter and decreased by making it longer. A single pipe, however, will also resonate at a frequency that produces any multiple of 1/2 wavelengths within its length. Producing resonance at the higher frequencies requires “overblowing” the pipe, a phenomenon which many of us remember when trying to play a child’s flute. If you blow too hard, the frequency increases to the second and successive resonances each double (an octive) above the previous in tone.
The fundamental frequency of an organ pipe with one end closed or sealed will be the frequency at which the pipe contains 1/4 wavelength. This will be 1/2 the frequency for the same length pipe with open ends. Successive resonances will occur at frequencies that produce any odd multiple of the fundamental 1/4 wavelength within the pipe as shown below.
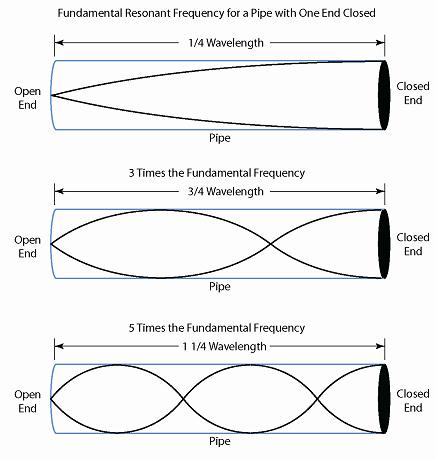
The 1/4 wavelength phenomenon occurs because as the sound wave (produced at the open end of the pipe) reflects from the closed end, its phase is reversed by 180 degrees. Recheck the above illustrations to understand this effect.
Resonance caused by multiple modes of vibration will be discussed in an upcoming blog.
– FJF –

 Water – De-ionized – Hints
Water – De-ionized – Hints  A Fond Farewell to John Fuchs
A Fond Farewell to John Fuchs  Millipore Testing – Evaluation by Particle Counting
Millipore Testing – Evaluation by Particle Counting  Tape Test for Cleaning Revisited
Tape Test for Cleaning Revisited